
Review |
 |
|
| Plane Mirrors | Concave Mirrors | Convex Mirrors | |||||||||||||||||||||||||
| shape | flat mirror | "caved in" | "bowed out" | ||||||||||||||||||||||||
| radius | R 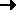 ¥
¥ |
R = 2f | R = 2f | ||||||||||||||||||||||||
position of principal focus |
- - - - | F is "in front of the mirror" | F is "behind of the mirror" | ||||||||||||||||||||||||
| image position |
di = - do | 1/do + 1/di = 1/f do is always positive di is positive if real di is negative if virtual f is positive if concave |
1/do + 1/di = 1/f do is always positive di is negative if virtual f is negative if convex |
||||||||||||||||||||||||
| image
properties |
virtual upright M = 1 |
six case ray
diagrams image varies with do
|
ray diagrams virtual, upright, M < 1
|
||||||||||||||||||||||||
| magnification | M = 1 | M = | di / do | = | I / O | | M = | di / do | = | I / O | | ||||||||||||||||||||||||
| rays | line up the top/bottom of the eye with the image, draw in a line - solid in the air, dotted behind the mirror, bounce it back to the object. Then put arrows pointing away from the object, to the mirror, and then into the eye. | #1 top of
the object, parallel to the axis, strikes the mirror, reflects through F #2 top of the object, through C, strikes the mirror, reflects back through C #3 top of the object, passes through F, strikes the mirror, reflects parallel to the axis |
#1 top of
the object, parallel to the axis, strikes the mirror, lines up with F, reflects back (dot in line to F) #2 top of the object, aims for C, strikes the mirror, reflects back (dot in line to C) #3 top of the object, aims for F, strikes the mirror,
reflects parallel to the axis |
||||||||||||||||||||||||
| multiple images of angled plane mirrors | N = (360º / q) - 1 | drop a normal to the first subscripted mirror measure di = - do draw in the standard rays |
- - - - |
| Copyright © 1997-2025 Catharine H. Colwell All rights reserved. PhysicsLAB Mainland High School Daytona Beach, FL 32114 |