#5 A body oscillates in SHM according to the equation: x = 5.0 cos (0.40t
+ 0.10)
A = 5.0 m
2pf = 0.40
f = 0.40/2p
f = 0.0637 hz
phase = 0.10 radians
x = 5.0 cos [0.40(2) + 0.10]
x = 3.11 m
(remember that your calculator must be in radians!]
#6 A body oscillates in SHM according to the equation: x = 8.0
cos (1.2t + 0.4)
A = 8.0 m
2pf = 1.2
f = 1.2/2p
f = 0.191 hz
T = 1 / f
T = 5.24 seconds
#8 Given: v = 1.57 m/sec; A = 0.5 mm = 0.0005
meters. Set v = A(2pf) and solve for the
frequency, f.
v= A(2pf)
1.57 = 0.0005 (2pf)
f = 1.57 / 0.0010p
f = 1.57 / 0.0010p
f = 500 hz
#17 Given: m = 0.20 grams = 0.0002 kg; f = 18
hz. Use the equations T = 1 / f and T = 2p
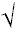 (m/k) and solve for k. (m/k) and solve for k.
1/18 = 2p 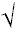 (0.0002/k) (0.0002/k)
k = 2.56 N/m
#18 Given: m = 5 kg, k = 50 N/m, A = 10 cm = 0.10 m. Use
conservation of energy, PEe = KE and solve for v.
KE = PEe
½mv2 = ½kA2
v = A 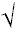 (k / m) (k / m)
v = 0.10 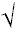 (50 / 5) (50 / 5)
v = 0.316 m/sec
#22 At 5.0 cm, the energy is both KE and PEe. By conservation of energy, PEe10
cm = (KE + PEe )5 cm
PEe = KE + PEe
½(50)(0.10)2 = ½(50)(0.05)2 +
½(5)v2
0.25 = 0.0625 + 2.5v2
0.1875 = 2.5 v2
v = 0.274 m/sec
|